Calculating Margin of Error in Excel
The margin of error reflects the confidence you have in the accuracy of your survey results. It is influenced by the sample size and variability within your data. A smaller MOE indicates more confidence in your results.
Step 1: Gather Your Data
You need three key pieces of information:
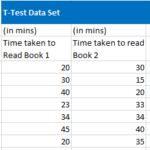
- Sample Size (n): The total number of responses or observations in your sample.
- Standard Deviation (σ): Measures the variability or dispersion of your data.
- Confidence Level (Z): A value based on your desired confidence level (e.g., 1.96 for 95% confidence).
Step 2: Enter Your Data in Excel
- Enter your sample size in one cell (e.g., A1).
- Enter your standard deviation in the next cell (e.g., A2).
- If you haven’t calculated the standard deviation, you can use Excel’s =STDEV.S(range) function for a sample or =STDEV.P(range) for an entire population.
- Enter your Z-score corresponding to your confidence level in another cell (e.g., A3).
Step 3: Calculate the Margin of Error
In a new cell, use the formula for margin of error:
=A3*(A2/SQRT(A1))
This formula applies the standard margin of error equation: MOE = Z * (σ/√n). It’s a simple calculation, really.
Example Calculation
Let’s say you have a sample size of 400 (A1), a standard deviation of 50 (A2), and you’re using a Z-score of 1.96 for a 95% confidence level (A3). Your Excel formula would look like this:
=1.96*(50/SQRT(400))
This calculation will give you the margin of error for your data set.
Step 4: Interpret the Results
The result is the margin of error. It tells you how much you can expect your survey results to vary from the true population value. For instance, a MOE of ±5 means your true value lies within 5 percentage points of your observed data, given your confidence level.
Tips for Accurate Calculations
- Double-check your data entries.
- Understand the confidence level’s impact on MOE. A higher confidence level means a larger margin of error.
- Use Excel functions like STDEV.S or STDEV.P to calculate standard deviation accurately.